[Algebra] Product of Two Negative Numbers
?By: Tao Steven Zheng (鄭濤)
【Problem】
Prove why the product of two negative real numbers is a positive real number.
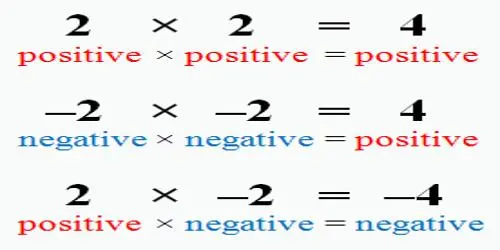

【Solution】
Let ? be two positive real numbers; subsequently,
and
?are their respective additive inverses.
A clever way to prove that is to begin by considering the equation
and then use this equation to show that and
.
First, factor out from the expression
:
Since ,
Thus,
Now, with the original equation, factor out ?from the expression
:
Thus,
Since? and
, we discover that?
Therefore, the product of two negative numbers is positive.
標(biāo)簽:

